Assigning Quantum Numbers
The following relationships involving the three quantum numbers come from the solution of the Schrödinger wave equation for the Hydrogen atom. In this solution, the values of the quantum numbers are fixed in the order listed.
- The first number to be fixed is the principal quantum number, n, which may only have a positive, nonzero integral value.
n = 1, 2, 3, 4, . . . - Second is the orbital angular momentum quantum number, L (instead of cursive l), which may be zero ro a positive integer, but not larger than n - 1 (n being the principal quantum number)
L = 0, 1, 2, 3, 4, . . . , n - 1 - Third is the magnetic quantum number, Ml, which may be a negative or positive integer, including zero, and ranging from -L to +L (where L is the orbital angular momentum quantum number).
Ml = -L, -L + 1, . . . , -2, -1, 0, 1, 2, . . . , L - 1, L
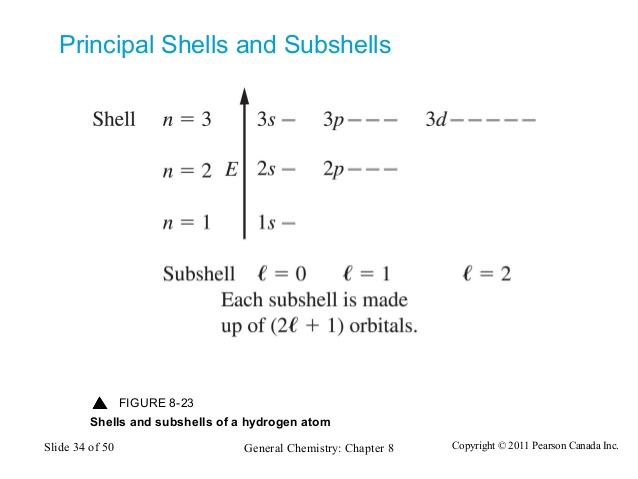
Principal Shells and Subshells
All orbitals with the same value of n are in the same principal electronic shell or principal level.
All orbitals with the same N and L values are in the same subshell.
Principal electronic shells are numbered according to the value of n. The first principal shell consists of orbitals with n = 1; the second principal shell of orbitals with n = 2; and so on. The value of n relates to the energy and most probable distance of an electron from the nucleus.
The higher the value of n, the greater the electron energy and the farther the electron is from the nucleus. Therefore, the principal quantum number has a physical significance. It defines how far away the electron is.
The quantum number L determines the angular distribution, or shape, of an orbital and Ml determines the orientation of the orbital.
The number of subshells in a principal electronic shell is the same as the number of allowed values of L. In the first principal shell (n = 1), there is only one possible L value: 0. Therefore, there's only one subshell. So, to generalize: the n'th principal electronic shell has n subshells.
The name given to a subshell depends on the value of the L quantum number.
First four subshells:
s subshell: L = 0, p subshell: L = 1
d subshell: L = 2, f subshell: L = 3
The number of orbitals are equal to the possible values of Ml, so -L, -L + 1 ... L-1 , L . That means that there are 2L + 1 orbitals in a subshell.
First four orbitals:
note: the lettering is the same, so the L values are equal
s orbital: 1 s orbital in an s subshell (2L + 1 = 1)
p orbital: 3 p orbitals in a p subshell (2L + 1 = 3)
d orbital: 5 d orbitals in a d subshell (2L + 1 = 5)
f orbital: 7 f orbitals in a f subshell (2L + 1 = 7)
To designate the particular principal shell, we sue a combination of a number and a letter. For example, the symbol 2p is used to mean the 2nd principal electronic shell and the p subshell and any of the three p orbitals.